Reassessing Free-Testosterone Calculation by Liquid Chromatography–Tandem Mass Spectrometry Direct Equilibrium Dialysis

[madman]

Context: Assessment of free testosterone (FT) might help evaluate androgen status in patients with borderline total testosterone (T) and/or altered sex hormone–binding globulin (SHBG) levels. However, the validity of different methods to measure FT is debatable.

Methods: Serum from 183 women and 146 men was analyzed using equilibrium dialysis (ED), with FT directly measured by liquid chromatography–tandem mass spectrometry. FT calculation was reevaluated for the mass action law–based equation according to Vermeulen (cFT-V), empirical equations according to Ly (cFT-L), and a proposed calculation based on a multistep, dynamic,allosteric model according to Zakharov (cFT-Z).

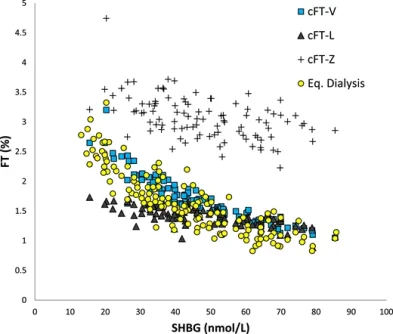
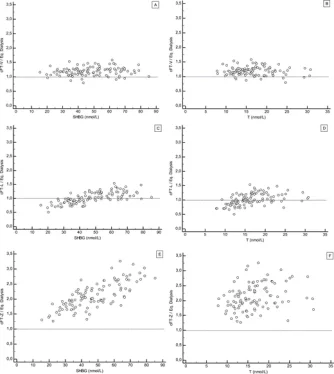
Results: FT level analyzed by ED [median,13 pmol/L (1.2% of T) in women; 248 pmol/L (1.5% of T) in men] was strongly inversely correlated to SHBG level, significantly to albumin level in women, and only weakly to SHBG level in men. The median [percentile (p) range, 2.5 to 97.5] ratios of calculated FT (cFT) over ED-FT (from European Male Aging Study samples) were 1.19 (0.9 to 1.47), 1.00 (0.69 to 1.42), and 2.05 (1.26 to 3.26) for cFT-V, cFT-L, and cFT-Z, respectively. The ratio for cFT-V was not significantly affected by SHBG, T, or albumin levels (p range, 0.17 to -0.01); ratios for cFT-L and cFT-Z were affected (P < 0.05 and P < 0.001, respectively) and strongly correlated with SHBG levels (p = 0.72 and 0.75, respectively). Rank correlations between cFT% and ED-FT% (for men) were 0.62, 0.74, and 0.89 for cFT-Z, cFT-L, and cFT-V, respectively.


Conclusion: FT results by direct ED confirm prior FT data from indirect ED and ultrafiltration methodologies. Calculations have inherent limitations, with clinically important differences among evaluated equations: cFT-V, although overestimating FT level, appears the most robust approximation, largely independent of SHBG, albumin, and T levels. (J Clin Endocrinol Metab103: 2167–2174, 2018)

 

[tareload]

Surprised no additional discussion on this one. Vermeulen hanging in there vs other methods.

Calculated estimations of FT

FT level was calculated from total T, SHBG and albumin serum levels according to the three following methods: (1) an equation based on the law of mass action as published by Vermeulen et al. (8) (cFT-V); (2) two empirically derived formulae (for men, the formula for T > 5 nM was used; for women, the formula <5 nM was used) as published by Ly and Handelsman (15) (cFT-L); and (3) according to a calculation based on a multistep, dynamic, allosteric model of testosterone binding to SHBG as published by Zakharov et al. (17) (cFT-Z). The values for cFT-Z from the EMAS samples (calculated with original T and SHBG from EMAS) were provided by Dr. R. Jasuja, Boston, MA, to the EMAS investigators. We had no direct access to the algorithm for cFT-Z; therefore, we were unable to present any data on cFT-Z for the samples from SIBLOS and from women.



Calculated estimates of FT

The cFT-Z values reported here have been supplied by the authors who reported data for cFT-Z in the EMAS cohort in the publication describing their multistep, dynamic, allosteric model to calculate FT (17). We requested access to the cFT-Z algorithm from the research group that developed this allosteric model algorithm. However, at the time of completion of this work, we had not been able to gain direct access to the algorithm. Therefore, it was not possible to make comparisons with cFT-Z for all three cohorts. This is a limitation of the current study that is beyond our control. We felt it important to evaluate cFT-Z in the current study because the results obtained by the authors according to their allosteric model to replicate the dimeric binding of T to SHBG differed substantially from the model based on the law of mass action (4, 17). Using the allosteric model, they reported higher FT% in men of 3% to 5% and that cFT-V substantially underestimated FT compared with their findings for FT by dialysis (17). Our results for the EMAS samples, indeed, do reproduce their finding that cFT-Z values are markedly higher than cFT-V values. Similarly, cFT-Z values are much higher compared with cFT-L. However, in contrast to their findings, our results also show that cFT-Z is markedly higher (about double) compared with FT measured by direct ED. Moreover, accuracy of cFT-Z as reflected in the ratio of cFT-Z over measured FT was strongly dependent on serum SHBG levels and, to a lesser degree, on T and albumin levels. At present, it is unclear what underlies the apparent discrepancy between the results reported by Zakharov et al. (17) and the findings in the current study performed on a same set of samples. A factor involved may be differences in ED methods between laboratories giving discrepant measured FT results. The descriptive nature of this study does not allow us to address possible merits or demerits of basic assumptions on which the allosteric model is based.

 

[tareload]

Free Testosterone: Pumping up the Tires or Ending the Free Ride?

The review by Goldman et al. (1) is a strong restatement of the free hormone hypothesis (FHH) with its contingent focus on measurement of so-called “free”

academic.oup.com

Widely used model-based formulae by Södergård et al. (21), Vermeulen et al. (22), and Mazer (23) incorporate two major sources of potential systematic error: assumptions about the stoichiometry and the binding affinity of testosterone for SHBG. Although the review attributes the inaccuracies of model-based formulae to their “linearity,” the discrepancies arise for a different reason: the mistaken assumptions on the stoichiometry of testosterone binding to SHBG. Prior to the crystal structure of SHBG in 2001 (24), the SHBG homodimer was believed to bind only a single testosterone molecule at dimerization junction (25), an assumption incorporated into all model-based FT formulae. However, the SHBG crystal structure showed that each monomer had a testosterone binding pocket so that the homodimer binds two, not one, testosterone molecules. Correcting this erroneous stoichiometry incorporated into the model-based binding equations reduces the marked deviations of model-based formulae from laboratory measured FT (26, 27). Another untested assumption of model-based formulae is the substitution of gravimetric measurement of SHBG by immunoassay—which differ between immunoassay methods—for SHBG binding capacity, which the binding equations actually invoke. Finally, the impact of genetic polymorphisms or acquired disease-related changes in SHBG binding affinity cannot be incorporated into the model-based FT formulae.

The review highlights a biophysical model by Zakharov et al. (28) for testosterone binding to SHBG with a novel but incompletely disclosed formula, patented by review coauthors and not yet subject to independent evaluation. The Zakharov biophysical model is a refinement of the equilibrium binding models by fitting two allosterically linked binding sites with distinct affinities, instead of two binding sites with the same affinity per SHBG monomer. Hence, although this work refines the understanding of the molecular binding of testosterone to SHBG, this minor variant of a model-based formula does not overcome the limitation of an obligatory requirement for plug-in binding affinities. Improvement by this thermodynamic model is at least partly attributable to its incorporation of the correct testosterone binding stoichiometry to SHBG. More troublesome problems arise for model-based FT formulae in their requirement for plug-in binding affinities of testosterone for SHBG, which are highly influential and responsible for much of the deviations of model-based formulae from laboratory-measured FT. Measuring such binding affinities, assumed to be population-wide and invariant, is as exacting as dialysis-based direct FT measurement; yet there is a fivefold variation among such estimates used in various equations (23).

The alternative, assumption-free fully empirical equations are given scant coverage denying them a fair hearing. These circumvent pitfalls arising from the assumptions requiring use of plug-in estimates for testosterone’s stoichiometry and binding affinity to SHBG. The fully empirical equations are created by regression of dialysis-based laboratory measured FT on measured serum testosterone and SHBG in the same samples using large databases, a methodology open to updating with new data. The most recent empirical formulae derived from a large data set (>4000 serum samples) has been subsequently verified in a different large data set (>2000 serum samples) from another laboratory (27, 29). In both studies, direct head-to-head testing showed that the model-based formulae consistently overestimate FT (27, 29, 30), a finding confirmed independently by others (26, 31–34). Curiously, Zakharov FT calculations produce even higher results than the Vermeulen equation making further independent evaluations of its validity essential. In that context, it is premature for the review to cite a lower confidence limit for calculated FT when the studies on which it is based have given rise to widely differing reference ranges for serum testosterone having reported a lower 2.5th centile of 348.3 ng/dL in one report (35) but subsequently as a 40% lower one (209 ng/dL) (36).

 

[tareload]

Free Testosterone: Pumping up the Tires or Ending the Free Ride?

The review by Goldman et al. (1) is a strong restatement of the free hormone hypothesis (FHH) with its contingent focus on measurement of so-called “free”

academic.oup.com

Widely used model-based formulae by Södergård et al. (21), Vermeulen et al. (22), and Mazer (23) incorporate two major sources of potential systematic error: assumptions about the stoichiometry and the binding affinity of testosterone for SHBG. Although the review attributes the inaccuracies of model-based formulae to their “linearity,” the discrepancies arise for a different reason: the mistaken assumptions on the stoichiometry of testosterone binding to SHBG. Prior to the crystal structure of SHBG in 2001 (24), the SHBG homodimer was believed to bind only a single testosterone molecule at dimerization junction (25), an assumption incorporated into all model-based FT formulae. However, the SHBG crystal structure showed that each monomer had a testosterone binding pocket so that the homodimer binds two, not one, testosterone molecules. Correcting this erroneous stoichiometry incorporated into the model-based binding equations reduces the marked deviations of model-based formulae from laboratory measured FT (26, 27). Another untested assumption of model-based formulae is the substitution of gravimetric measurement of SHBG by immunoassay—which differ between immunoassay methods—for SHBG binding capacity, which the binding equations actually invoke. Finally, the impact of genetic polymorphisms or acquired disease-related changes in SHBG binding affinity cannot be incorporated into the model-based FT formulae.

The review highlights a biophysical model by Zakharov et al. (28) for testosterone binding to SHBG with a novel but incompletely disclosed formula, patented by review coauthors and not yet subject to independent evaluation. The Zakharov biophysical model is a refinement of the equilibrium binding models by fitting two allosterically linked binding sites with distinct affinities, instead of two binding sites with the same affinity per SHBG monomer. Hence, although this work refines the understanding of the molecular binding of testosterone to SHBG, this minor variant of a model-based formula does not overcome the limitation of an obligatory requirement for plug-in binding affinities. Improvement by this thermodynamic model is at least partly attributable to its incorporation of the correct testosterone binding stoichiometry to SHBG. More troublesome problems arise for model-based FT formulae in their requirement for plug-in binding affinities of testosterone for SHBG, which are highly influential and responsible for much of the deviations of model-based formulae from laboratory-measured FT. Measuring such binding affinities, assumed to be population-wide and invariant, is as exacting as dialysis-based direct FT measurement; yet there is a fivefold variation among such estimates used in various equations (23).

The alternative, assumption-free fully empirical equations are given scant coverage denying them a fair hearing. These circumvent pitfalls arising from the assumptions requiring use of plug-in estimates for testosterone’s stoichiometry and binding affinity to SHBG. The fully empirical equations are created by regression of dialysis-based laboratory measured FT on measured serum testosterone and SHBG in the same samples using large databases, a methodology open to updating with new data. The most recent empirical formulae derived from a large data set (>4000 serum samples) has been subsequently verified in a different large data set (>2000 serum samples) from another laboratory (27, 29). In both studies, direct head-to-head testing showed that the model-based formulae consistently overestimate FT (27, 29, 30), a finding confirmed independently by others (26, 31–34). Curiously, Zakharov FT calculations produce even higher results than the Vermeulen equation making further independent evaluations of its validity essential. In that context, it is premature for the review to cite a lower confidence limit for calculated FT when the studies on which it is based have given rise to widely differing reference ranges for serum testosterone having reported a lower 2.5th centile of 348.3 ng/dL in one report (35) but subsequently as a 40% lower one (209 ng/dL) (36).

Calculate Free Testosterone with TruT by FPT

[0026] Fig. 7 depicts the thermodynamic Parameters associated with testosterone's binding to SHBG derived from the fit of binding isotherms and ITC data to new model. While this parameter set is not unique, together they consistently describe the binding isotherms, depletion curves and ITC data...

www.excelmale.com